zenpundit.com

[ by Charles Cameron — omega worms in science and scripture ]
.

As regular readers know, I am interested in Omega — the End, in “alpha and omega” terms — so I was naturally intrigued by this tweet, with Adam Elkus kindly put in my twitter feed and those of others who follow him:

If you are a worm scientist and know what a "omega turn" is, please help fill out our survey! https://t.co/a0JggSHEiH

— OpenWorm (@OpenWorm) June 7, 2016

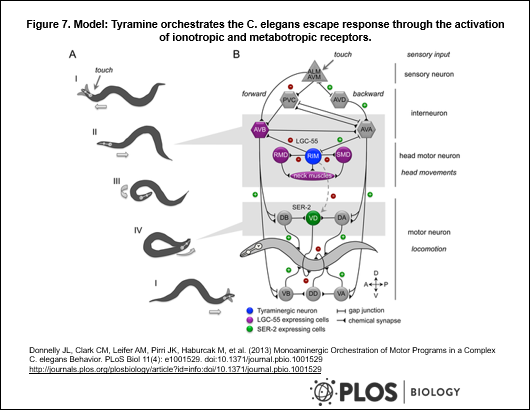
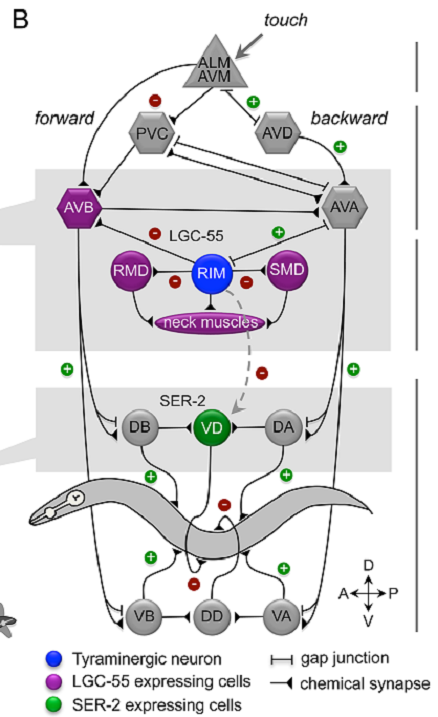
I’m not a “worm scientist” but wanted to know what an omega turn is, so I browsed around a bit and found this diagram:

Donnelly et al, Monoaminergic Orchestration of Motor Programs in a Complex C. elegans Behavior

**

Now please don’t imagine I know what that means to a worm scientist — I was expecting something more like the worm in the lowest section of thIs Beatus Apocalypse:

or maybe this:

from a different Beatus manuscript, where it appears the worm has turned quite a few times.

**

In the unlikely event that I should attempt a translation of St John‘s revelatory vision on the Isle of Patmos into science fiction, be assured that I shall include a reference to that image among my illustrations, with a footnote perhaps, pointing to Mark 9.48:

Where their worm dieth not, and the fire is not quenched.

Today, however, my appreciation for all things apocalyptic must give way to another interest of mine, that of the pervasive use of [node and edge] graphs in our contemporary world. Here again is the central column of that image:

It interests me here as yet another illustration of the degree to which graphs serve as a fundamental substrate of our understanding of the world — and hence my continuing interest in their use in game board design — both of which I’ve been exploring in other posts in this series:

On the felicities of graph-based game-board design: preliminaries

On the felicities of graph-based game-board design: two dazzlers

On the felicities of graph-based game-board design: three

On the felicities of graph-based game-board design: four

On the felicities of graph-based game-board design: five

On the felicities of graph-based game-board design: six

On the felicities of graph-based game-board design: seven

**

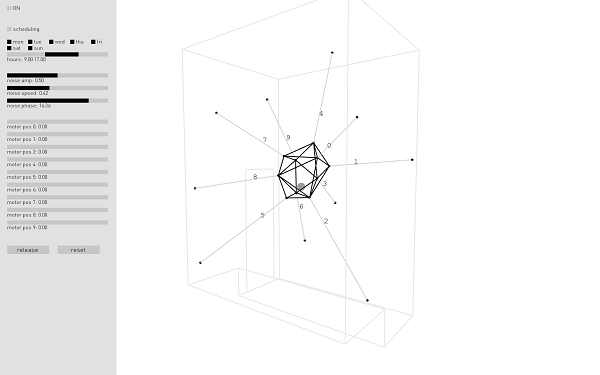
After the glamour of Beatus manuscripts and PLOS worm diagrams, it may seem almost a let down to turn to irregular polyhedra — but the move from two-dimensional graphs to their three-dimensional cousins is a short one, and since we live in what at least appears to be a (spatially) three-dimensional world, one which should also be considered in terms of game board and concept-modelling design.

The following illustration —

— and accompanying video, from Filip Visnjic, Irregular Polyhedron Study #1 – Vertex, edge and volume, may accordingly be of interest:

Irregular Polyhedron Study #1 from Bjørn Gunnar Staal on Vimeo.

[ by Charles Cameron — Robert Redford and Brad Pitt on a Berlin rooftop ]
.

how do you draw a circle in an entirely linear medium?

**

The movie is Spy Game, with Robert Redford and Brad Pitt.

To my mind, it’s a brilliant piece of film making: director Tony Scott chose a terrific location for Nathan Muir (Redford)’s debrief reaming of Tom Bishop (Pitt), in the course of which Muir very pointedly tells Bishop:

Listen to this, because this is important. If you’d pulled a stunt there and got nabbed, I wouldn’t come after you. You go off the reservation, I will not come after you.

That’s the heart of the movie, right there, in negative — because the whole movie is about Bishop going off reservation in China, pulling a stunt there, and getting nabbed by the Chinese, and Muir coming after Bishop and rescuing him, with great shenanigans and flashbacks along the way.

Scott wants to draw a circle around that point, to drive it home — but this is a movie, a totally linear sequence frames, whether celluloid or digital, so how do you draw a circle in a linear medium?

Scott shoots the scene atop a circular roof, and before, during and after the conversation between the two men, has the camera circle the building:

**

I know, I stretch the limits of this blog mercilessly — and I’m spending this post on a piece of cinema technique. Let’s just say that I take Adam Elkus‘ words seriously:

Clausewitz himself was heavily inspired by ideas from other fields and any aspiring Clausewitzian ought to mimic the dead Prussian’s habit of reading widely and promiscuously.

I’m being promiscuous.

**

There are two other major points caught in Scott’s tight circle. One offers the essence of Spy Game, emphasis on the spy:

Bishop: Okay, help me understand this one. Nathan, what are we doing here? Don’t bullshit me about the greater good.
Muir: That’s exactly what it’s about. Because what we do is, unfortunately, very necessary.

The other gets to the other half of the name Spy Game — game:

Bishop: It’s not a fucking game!
Muir: Yes, it is. That’s exactly what it is. It’s no kid’s game, either, but a whole other game. And it’s serious, and it’s dangerous, and it’s not one you want to lose.

So, in the gospel according to Spy Game, espionage is a deadly and death-dealing game, played unfortunately but very necessarily for the greater good. All that in three short minutes, with a circle drawn around it for emphasis.

**

Thus a problem in geometry is artfully transcended.

[ by Charles Cameron — something’s closing in on us ]
.

**

Sources:

Adam Elkus, I was not kidding

Plain Dealer, Akron Art Museum rescues M.C. Escher from his reputation

[ by Charles Cameron — light-hearted, almost science-fictional “butterfly-hurricane” question in geopolitics, with an Elkus follow-up ]
.

A scene from the 2000 Florida recount: Palm Beach County’s canvassing board chairman eyes a questionable ballot as Republican attorney John Bolton looks on. Image: Greg Lovett/AP

**

Is the Islamic State an “unanticipated consequence” of Bush v Gore?

Donald Trump, as quoted in Vox’s America’s unlearned lesson: the forgotten truth about why we invaded Iraq:

You do whatever you want. You call it whatever you want. I want to tell you. They lied. They said there were weapons of mass destruction, there were none. And they knew there were none. There were no weapons of mass destruction.

Without getting too far into the weeds, my question is this:

Is it fair to say that the Islamic State (aka ISIS, ISIL) was born in 2006 in response to the American invasion and occupation of Iraq, which in turn was initiated by President George W Bush, who became Commander in Chief in 2000 in a disputed election only resolved by the Supreme Court’s ruling in Bush v. Gore, 531 U.S. 98 (2000)? And if so, looking back at the branching possibilities and eventualities that led to the creation of IS, might I plausibly suggest the Islamic State owes its very existence to a “hanging chad”?

If the Florida electoral votes hadn’t been disputed on account of flaws in the mechanical method by which they were registered, in other words, might there have been no invasion of Iraq, and hence no IS as such?

I know: this is hugely simplistic, both in terms of the election and of the drives behind Zarqawi and company — but I’m looking for an illustration of a very small digfference in “initial conditions” giving rise to a notable difference in a “later state” of a related aspect of the world system, Lorenz’s butterfly effect.

I understand that “dimpled chads” were also part of the “initial conditions” in question, but “hanging by a chad” works better as a phrase than “dimpled by a chad” — although “hanging by a dimple” has a certain charm.

Srsly, though — to what extent is our current timeline, in which IS may reasonably be viewed as a notable threat, causally connected to the resolution of a mechanical flaw in voting machine design?

**

And more seriously:

I very much appreciated Adam Elkus‘ post, Trump: The Explanation of No Explanation, and the great quote from Charles Kurzman on the Iranian Revolution from which Adam kicks off:

All of [the Iran] analyses are wrong, even if events unfold the way they predict. After all, if you make enough predictions, some are bound to look accurate. They are wrong because the outcome of this week’s events is simply unpredictable. Unpredictable means that no matter how well-informed you may be, it is impossible to know what will happen next. Moments of turmoil make a mockery of accumulated knowledge. Routine behavior, on the other hand, can be predicted. It is likely to occur tomorrow the way it occurred yesterday, with adjustments for shifts over time. But breaks from routine are a different beast altogether. The more that people feel that normal rules of behavior no longer hold, the more they search around for new rules, surveying their neighbors, collecting rumors, checking their text messages in a frantic attempt to figure out what everyone else is planning to do. Very few people are willing to be the only ones out in the street when the security forces start to advance. If people expect millions of their compatriots to demonstrate, many will want to help make history…. Such moments of mass confusion are unsettling and rare. They usually fade back into routine. Occasionally, however, they create their own new routines, even new regimes, as they did in 1978-1979. In later retelling of these episodes, especially by experts, confusion is often downplayed, as though the outcomes might have been known in advance. But that is not how Iranians are experiencing current events. Their experience, and their response to their experience, will determine the outcome.

[ by Charles Cameron — for Adam Elkus ]
.

A case study in the heliotrope:

Do we gain as much in science as we lose in poetry, when we switch explanatory frameworks?

**

F Scott Fitzgerald:

The test of a first-rate intelligence is the ability to hold two opposed ideas in mind at the same time and still retain the ability to function.

How about holding two explanatory frameworks in mind?

Adam, I think you’re doing something of the sort with qualitative & quantitative approaches, right? And I quote…

The work merges my longstanding interests in intellectual history and qualitative research approaches to studying strategy and decision-making and my technical interests in simulation, modeling, cognitive science, and machine intelligence programming.