Most intriguing game-theoretic comment of the year thus far
[ by Charles Cameron — at the intersection of zero-sum and non-zero sum games ]
.
And the hands-down winner is — opening today’s Washington Post to the op-ed page — President Hassan Rouhani of Iran, who says:
The world has changed. International politics is no longer a zero-sum game but a multi-dimensional arena where cooperation and competition often occur simultaneously. Gone is the age of blood feuds. World leaders are expected to lead in turning threats into opportunities.
I think he’s right, though I’ll leave the question of whether he means it TBD — but if he does, that’s a.. that’s a.. that’s a Major Game Changer — and verra interesting in any case:
**
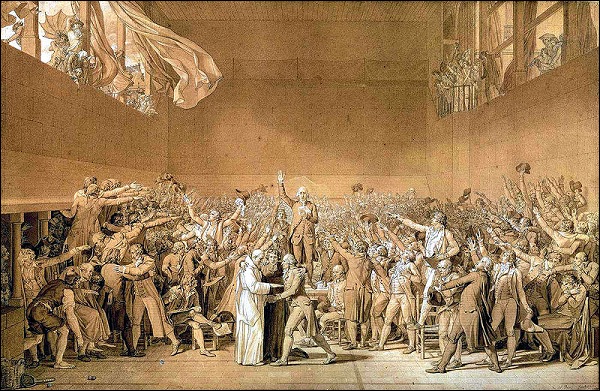
For your further edification, here’s what a genuine game-changer, in both literal and metaphoric sense of the phrase, looks like:
.
The court is a tennis court, the game in play is revolutionary politics, the event is the Tennis Court Oath, where the members of the National Assembly gathered to swear “not to separate, and to reassemble wherever circumstances require, until the constitution of the kingdom is established” — the drawing is by Jacques-Louis David.



September 20th, 2013 at 3:56 pm
International politics never has been a zero-sum game. The best diplomacy has always been win-win.
.
But somehow, since the Vietnam War or so, Americans have become convinced that might will always prevail, despite the evidence piling up that it’s more complicated than that.
.
It was a good thing for Rouhani to say. It’s easier for countries that have less power to say things like this, though, and it may be discounted for that reason.
.
And we need to see exactly how the nuclear negotiations go.
September 20th, 2013 at 5:03 pm
Hi Cheryl:
.
One of the problems with chess is that it’s a zero-sum game — and I tried to “rectify” that “omission” by adding a non-zero-sum element in my own (never played, as far as I know, but I still very much like the idea).
.
The thing is, complicated non-zero-sum games may have zero-sum games within them, no? As in: I’ll let you win this zero-sum game if you let me win that one? So then there’s the question of whether they’re separable, whether the braids can be disentangled? I’m wondering, ifn effect, whether there’s an interfacing between the two kinds of games that parallels the interfacing between laminar and turbulent flows at some game theoretic level — but I’ll never be the von Karman of games myself!
.
And then another idea crops up: does the transition from peace to war and back to peace again (the first via “continuation by other means” and the second via diplomatic negotiation of treaties) imply a shift in emphasis between non-zero-sum games (in peace) and zero-sum games (in war)? I’m no sure I’m expressing myself (ie “thinking”) clearly enough…
.
I was also wondering about the use of game-theoretic phrasing in such a public arena as the President of a nation addressing the US population via a Washington Post op-ed, and checked the White House pages to see if President Obama had used the term. Joe Biden seems to use it often enough, but the only Presidential use i could find on a hasty search was this, from January 30, 2009, quoted as an epigraph here:
If any of our readers has an OED handy, I’d be interested to know who first used the terms “zero-zum” and “non-zero-sum”, and when.
.
So much to learn, so few hours in the day… days in the life…
September 20th, 2013 at 8:11 pm
I don’t have a subscription to OED, but a google search for its etymology indicates that the term was first used by John Von Neumann in his 1944 book ‘Theory of Games and Behavior”
http://en.wikipedia.org/wiki/Theory_of_Games_and_Economic_Behavior
.
However a search on Wolfram Alpha leads to the 1921 Colonel Blotto games by Emile Borel
http://en.wikipedia.org/wiki/Blotto_games
September 20th, 2013 at 8:28 pm
A couple other early notable mentions:
.
Stafford Beer Decision and Control
http://goo.gl/GLffxM
.
G H Burgin On Playing Two-Person Zero-Sum Games against Nonminimax Players
http://goo.gl/C24KCL
September 20th, 2013 at 8:55 pm
No answers, but I’ll see you and raise you: Is it a zero-sum game if you think it’s a zero-sum game?
September 20th, 2013 at 9:10 pm
Grurrat:
.
Thanks! I’m clearly stretched over too many battlefields!
.
Cheryl:
.
Nice one: if you do, your strategy might depend on whether you believe it’s iterative…
September 21st, 2013 at 2:13 am
As for Von Neumann, I’ve read as much as I could understand, which wasn’t much past the introductory material. According to the index of my copy, he references the “zero-sum condition,” “zero-sum extension,” and “zero-sum restrictions.”
.
This is a book I’ve dabbled with and never mastered/completed, so I offer this only to add emphasis to comments already offered.
September 21st, 2013 at 6:56 pm
Rouhani’s notion — “International politics is no longer a zero-sum game but a multi-dimensional arena where cooperation and competition often occur simultaneously” — has been in play among theorists for some years now. I’ve not collected everything about it, but some background is discussed under the rubric of “strategic multiplexity” in two posts:
http://twotheories.blogspot.com/2008/12/strategic-multiplexity-another-trait-of.html
http://twotheories.blogspot.com/2012/09/strategic-multiplexity-revisited.html
Another way to look at it, following comments here, is that win-win (cooperative) and win-lose (competitive and conflictive) games are being played simultaneously, and interactively.
Apropos, here’s a recent statement by Naomi Klein disparaging the strategy of Big Green groups: “Their so-called win-win strategy has lost. That was the idea behind cap-and-trade. And it was a disastrously losing strategy. … The phrase win-win is interesting, because there are a lot of losers in the win-win strategy. A lot of people are sacrificed in the name of win-win. …”
September 21st, 2013 at 7:47 pm
Hi David:
.
Thanks!
.
Yes, “simultaneously, and interactively” is pretty much what I’d like to get a sense of, in some medium or other — I think my chess variant (mentioned in comment #2 above, in what looks like a truncated sentence) would go some way in that direction, but I’d take a Hokusai or van Gogh, Yeats or Blakean description of how it works too — or a mathematical model, even though i wouldn’t understand it unless someone could “translate” it into dynamic visuals on YouTube…
.
I think I’d originally intended to include a description of my chess variant in comment #2, as follows:
September 21st, 2013 at 8:36 pm
“One of the problems with chess is that it’s a zero-sum game —”
*
But then, if my memory hasn’t failed me, it only became a zero-sum game when the Knight was introduced into the game. Which I also believe happened around the time when Alexander the Great took over the Persian Empire.
*
So I think Iran would sort of know when the game has changed back again.
*
Which, in the “game”, the Knight goes after the elites, so if the “game” has changed, it means we have formed a consensus about the elites, which, above all else, is disproved here on the Zen.
*
Otherwise international politics is just about a bunch of Mongols.
*
Ask the Russians about them
September 23rd, 2013 at 2:28 pm
Does a zero-sum win end an iterated game?
Self-fulfilling prophecy:
: W. claims you cannot trust the government.
: W. becomes president.
: W. proves you cannot trust the government.
The precedent now dictates that the claim can never be disproved.
Consider Matkot, termed a ‘non-competitive game.’ As long as the game is iterating, the participants are winning. But ‘winning’ is a verb that comes to an end, so both participants know that at the end, they both lose. Meanwhile, non-participant neighbors take losses when they get trampled. The zero-sum is that the participants win, in that they get what they want, which is to play the game, while neighbors have losses imposed upon them.